In this article, we will discuss the uses and Applications of Bernoulli’s Principle. But before that, let us find out what Bernoulli’s Principle actually is.
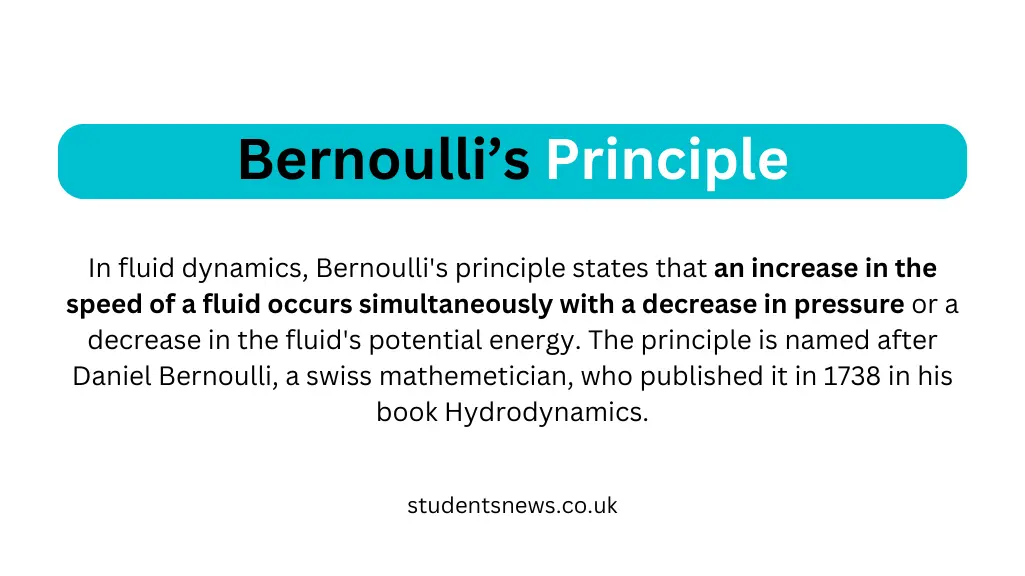
Bernoulli’s Principle is a key concept in fluid dynamics that helps explain how fluids behave when they flow. It’s named after Daniel Bernoulli, a Swiss mathematician. Simply put, the principle states that as the speed of a fluid increases, its pressure decreases. This relationship is crucial for understanding many things, like how airplanes fly or why your shower curtain gets pulled inwards when the water is running.
Here’s an easy way to think about it: imagine blowing air over a piece of paper. The faster air moving over the top of the paper causes the paper to lift. That’s Bernoulli’s Principle in action! This principle works because of the conservation of energy: the total energy in a fluid remains the same, so if a fluid speeds up, something else (like pressure) has to decrease.
Bernoulli’s Equation
Bernoulli’s Equation is a mathematical way to express this principle. It helps us calculate the relationship between a fluid’s pressure, speed, and height. The equation looks like this:
P + ½ρv² + ρgh = constant
It might look complicated, but here’s what it means:
- P is the pressure in the fluid.
- ρ (rho) is the density of the fluid.
- v is the fluid’s velocity (how fast it’s moving).
- g is gravity.
- h is the height of the fluid.
Using this equation, we can figure out how fast a fluid is moving or how much its pressure has changed. It’s especially useful in applications like airplane wings or measuring how fast water flows through a pipe.
Key Applications of Bernoulli’s Principle
Bernoulli’s Principle is all around us! Let’s take a look at some common applications:
- Aerodynamics: One of the most famous applications is how airplane wings generate lift. The air moves faster over the top of the wing, lowering the pressure, while the slower-moving air underneath creates higher pressure. This difference in pressure lifts the plane!
- Fluid Flow Measurement: Tools like the Venturi meter use Bernoulli’s Principle to measure the flow rate of liquids. The fluid speeds up as it passes through a narrower section, which helps calculate the flow.
- Medical Applications: Bernoulli’s Principle helps explain blood flow in the body. For example, when arteries narrow, blood speeds up, causing a drop in pressure. This can be a sign of health issues.
- Everyday Devices: Have you ever used a perfume spray? That’s Bernoulli’s Principle in action! When you squeeze the bottle, air moves quickly over the liquid, creating low pressure that draws the liquid out as a mist.
The table below highlights the advantages and disadvantages of using Bernoulli’s Principle in these applications:
| Advantages | Disadvantages |
|---|---|
|
|
Advanced Applications
Bernoulli’s Principle isn’t just limited to basic uses like airplanes and spray bottles. It also plays a huge role in more advanced fields:
- Wind Turbines: Engineers use Bernoulli’s Principle to study how air flows around wind turbine blades. This helps them design turbines that capture more wind energy efficiently.
- Acoustics: The principle is used to explain how sound waves travel through air and water. It’s also used in the design of musical instruments and sound systems.
- Compressible Fluids: While Bernoulli’s Principle usually applies to incompressible fluids, it can be modified to work with compressible fluids like air in specific cases, such as gas pipelines or air compressors.
These applications show how versatile Bernoulli’s Principle can be when applied creatively!
Limitations of Bernoulli’s Principle
Even though Bernoulli’s Principle is widely used, it does have its limitations. Here are some key points to consider:
- Only works with incompressible fluids: It’s not accurate when used with fluids that can compress, like gases at high speeds.
- Assumes steady flow: Bernoulli’s Principle only applies when the fluid is flowing smoothly and steadily. If the flow changes rapidly, the principle breaks down.
- Ignores friction: Real-world fluids have friction and viscosity, which Bernoulli’s Principle doesn’t take into account. This can lead to errors when applied to real systems like pipes or turbines.
It’s important to remember these limitations when using Bernoulli’s Principle in more complex situations.
Misconceptions About Bernoulli’s Principle
There are some common misunderstandings about Bernoulli’s Principle. Let’s clear a few of them up:
- Misconception 1: It’s the only reason airplanes fly: While Bernoulli’s Principle explains part of how airplanes generate lift, it’s not the whole story. The shape of the wing and other forces also play a big role.
- Misconception 2: It explains the Magnus Effect: The Magnus Effect, which explains why spinning objects like soccer balls curve, involves more than just Bernoulli’s Principle. Rotation and other forces are also involved.
By understanding these misconceptions, we can better appreciate the true power and limitations of Bernoulli’s Principle.
Conclusion
Bernoulli’s Principle is a key concept in fluid dynamics that explains how the speed and pressure of a fluid are related. From airplane wings to wind turbines, it has countless applications in science and engineering.
Although it has its limitations, especially with compressible fluids and turbulent flows, it remains a powerful tool for understanding how fluids move. As we’ve seen, its uses span from everyday devices like spray bottles to advanced technologies like wind turbines and acoustic systems. Understanding Bernoulli’s Principle helps us see the hidden forces at work all around us!
